...
Matriz
RESUMO: A definição do arquivo de entrada segue a configuração matricial adotada por Taillard (1993), apresentada como:
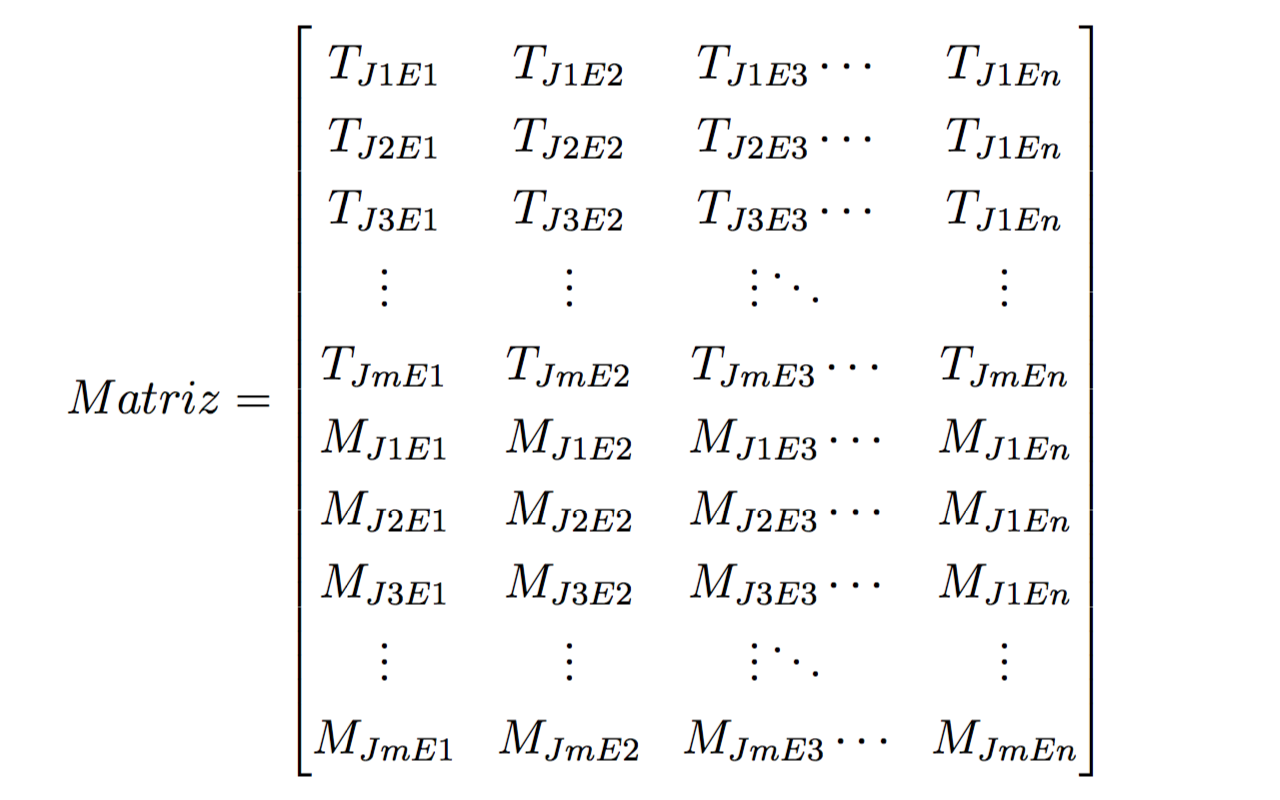
a matriz de dados é subdividida em tempos (TJmEn) e maquinas (MJmEn). Onde T representa o tempo da operação, J o job, E a etapa do roteiro. Essa configuração permite uma interpretação compacta do roteiro
de fabricação para cada job e seus respectivostempos de processamento.
Adams, Balas and Zawack
| Instance | Jobs | Machines | Lower bound | Upper bound |
|---|---|---|---|---|
| abz5 | 10 | 10 | 1234 | 1234 |
| abz6 | 10 | 10 | 943 | 943 |
| abz7 | 20 | 15 | 656 | 656 |
| abz8 | 20 | 15 | 648 | 665 |
| abz9 | 20 | 15 | 678 | 678 |
Fisher and Thompson
| Instance | Jobs | Machines | Lower bound | Upper bound |
|---|---|---|---|---|
| ft06 | 6 | 6 | 55 | 55 |
| ft10 | 10 | 10 | 930 | 930 |
| ft20 | 20 | 5 | 1165 | 1165 |
Lawrence
| Instance | Jobs | Machines | Lower bound | Upper bound |
|---|---|---|---|---|
| la01 | 10 | 5 | 666 | 666 |
| la02 | 10 | 5 | 655 | 655 |
| la03 | 10 | 5 | 597 | 597 |
| la04 | 10 | 5 | 590 | 590 |
| la05 | 10 | 5 | 593 | 593 |
| la06 | 15 | 5 | 926 | 926 |
| la07 | 15 | 5 | 890 | 890 |
| la08 | 15 | 5 | 863 | 863 |
| la09 | 15 | 5 | 951 | 951 |
| la10 | 15 | 5 | 958 | 958 |
| la11 | 20 | 5 | 1222 | 1222 |
| la12 | 20 | 5 | 1039 | 1039 |
| la13 | 20 | 5 | 1150 | 1150 |
| la14 | 20 | 5 | 1292 | 1292 |
| la15 | 20 | 5 | 1207 | 1207 |
| la16 | 10 | 10 | 945 | 945 |
| la17 | 10 | 10 | 784 | 784 |
| la18 | 10 | 10 | 848 | 848 |
| la19 | 10 | 10 | 842 | 842 |
| la20 | 10 | 10 | 902 | 902 |
| la21 | 15 | 10 | 1046 | 1046 |
| la22 | 15 | 10 | 927 | 927 |
| la23 | 15 | 10 | 1032 | 1032 |
| la24 | 15 | 10 | 935 | 935 |
| la25 | 15 | 10 | 977 | 977 |
| la26 | 20 | 10 | 1218 | 1218 |
| la27 | 20 | 10 | 1235 | 1235 |
| la28 | 20 | 10 | 1216 | 1216 |
| la29 | 20 | 10 | 1152 | 1152 |
| la30 | 20 | 10 | 1355 | 1355 |
| la31 | 30 | 10 | 1784 | 1784 |
| la32 | 30 | 10 | 1850 | 1850 |
| la33 | 30 | 10 | 1719 | 1719 |
| la34 | 30 | 10 | 1721 | 1721 |
| la35 | 30 | 10 | 1888 | 1888 |
| la36 | 15 | 15 | 1268 | 1268 |
| la37 | 15 | 15 | 1397 | 1397 |
| la38 | 15 | 15 | 1196 | 1196 |
| la39 | 15 | 15 | 1233 | 1233 |
| la40 | 15 | 15 | 1222 | 1222 |
Applegate and Cook
| Instance | Jobs | Machines | Lower bound | Upper bound |
|---|---|---|---|---|
| la01 | 10 | 5 | 666 | 666 |
| la02 | 10 | 5 | 655 | 655 |
| la03 | 10 | 5 | 597 | 597 |
| la04 | 10 | 5 | 590 | 590 |
| la05 | 10 | 5 | 593 | 593 |
| la06 | 15 | 5 | 926 | 926 |
| la07 | 15 | 5 | 890 | 890 |
| la08 | 15 | 5 | 863 | 863 |
| la09 | 15 | 5 | 951 | 951 |
| la10 | 15 | 5 | 958 | 958 |
| la11 | 20 | 5 | 1222 | 1222 |
| la12 | 20 | 5 | 1039 | 1039 |
| la13 | 20 | 5 | 1150 | 1150 |
| la14 | 20 | 5 | 1292 | 1292 |
| la15 | 20 | 5 | 1207 | 1207 |
| la16 | 10 | 10 | 945 | 945 |
| la17 | 10 | 10 | 784 | 784 |
| la18 | 10 | 10 | 848 | 848 |
| la19 | 10 | 10 | 842 | 842 |
| la20 | 10 | 10 | 902 | 902 |
| la21 | 15 | 10 | 1046 | 1046 |
| la22 | 15 | 10 | 927 | 927 |
| la23 | 15 | 10 | 1032 | 1032 |
| la24 | 15 | 10 | 935 | 935 |
| la25 | 15 | 10 | 977 | 977 |
| la26 | 20 | 10 | 1218 | 1218 |
| la27 | 20 | 10 | 1235 | 1235 |
| la28 | 20 | 10 | 1216 | 1216 |
| la29 | 20 | 10 | 1152 | 1152 |
| la30 | 20 | 10 | 1355 | 1355 |
| la31 | 30 | 10 | 1784 | 1784 |
| la32 | 30 | 10 | 1850 | 1850 |
| la33 | 30 | 10 | 1719 | 1719 |
| la34 | 30 | 10 | 1721 | 1721 |
| la35 | 30 | 10 | 1888 | 1888 |
| la36 | 15 | 15 | 1268 | 1268 |
| la37 | 15 | 15 | 1397 | 1397 |
| la38 | 15 | 15 | 1196 | 1196 |
| la39 | 15 | 15 | 1233 | 1233 |
| la40 | 15 | 15 | 1222 | 1222 |
Storer, Wu and Vaccari
| Instance | Jobs | Machines | Lower bound | Upper bound |
|---|---|---|---|---|
| swv01 | 20 | 10 | 1407 | 1407 |
| swv02 | 20 | 10 | 1475 | 1475 |
| swv03 | 20 | 10 | 1398 | 1398 |
| swv04 | 20 | 10 | 1464 | 1464 |
| swv05 | 20 | 10 | 1424 | 1424 |
| swv06 | 20 | 15 | 1630 | 1671 |
| swv07 | 20 | 15 | 1513 | 1594 |
| swv08 | 20 | 15 | 1671 | 1752 |
| swv09 | 20 | 15 | 1633 | 1655 |
| swv10 | 20 | 15 | 1663 | 1743 |
| swv11 | 50 | 10 | 2983 | 2983 |
| swv12 | 50 | 10 | 2972 | 2977 |
| swv13 | 50 | 10 | 3104 | 3104 |
| swv14 | 50 | 10 | 2968 | 2968 |
| swv15 | 50 | 10 | 2885 | 2885 |
| swv16 | 50 | 10 | 2924 | 2924 |
| swv17 | 50 | 10 | 2794 | 2794 |
| swv18 | 50 | 10 | 2852 | 2852 |
| swv19 | 50 | 10 | 2843 | 2843 |
| swv20 | 50 | 10 | 2823 | 2823 |
Taillard
| Instance | Jobs | Machines | Lower bound | Upper bound |
|---|---|---|---|---|
| ta01 | 15 | 15 | 1231 | 1231 |
| ta02 | 15 | 15 | 1244 | 1244 |
| ta03 | 15 | 15 | 1218 | 1218 |
| ta04 | 15 | 15 | 1175 | 1175 |
| ta05 | 15 | 15 | 1224 | 1224 |
| ta06 | 15 | 15 | 1238 | 1238 |
| ta07 | 15 | 15 | 1227 | 1227 |
| ta08 | 15 | 15 | 1217 | 1217 |
| ta09 | 15 | 15 | 1274 | 1274 |
| ta10 | 15 | 15 | 1241 | 1241 |
| ta11 | 20 | 15 | 1357 | 1357 |
| ta12 | 20 | 15 | 1367 | 1367 |
| ta13 | 20 | 15 | 1342 | 1342 |
| ta14 | 20 | 15 | 1345 | 1345 |
| ta15 | 20 | 15 | 1339 | 1339 |
| ta16 | 20 | 15 | 1360 | 1360 |
| ta17 | 20 | 15 | 1462 | 1462 |
| ta18 | 20 | 15 | 1377 | 1396 |
| ta19 | 20 | 15 | 1332 | 1332 |
| ta20 | 20 | 15 | 1348 | 1348 |
| ta21 | 20 | 20 | 1642 | 1642 |
| ta22 | 20 | 20 | 1561 | 1600 |
| ta23 | 20 | 20 | 1518 | 1557 |
| ta24 | 20 | 20 | 1644 | 1644 |
| ta25 | 20 | 20 | 1558 | 1595 |
| ta26 | 20 | 20 | 1591 | 1643 |
| ta27 | 20 | 20 | 1652 | 1680 |
| ta28 | 20 | 20 | 1603 | 1603 |
| ta29 | 20 | 20 | 1573 | 1625 |
| ta30 | 20 | 20 | 1519 | 1584 |
| ta31 | 30 | 15 | 1764 | 1764 |
| ta32 | 30 | 15 | 1774 | 1784 |
| ta33 | 30 | 15 | 1788 | 1791 |
| ta34 | 30 | 15 | 1828 | 1829 |
| ta35 | 30 | 15 | 2007 | 2007 |
| ta36 | 30 | 15 | 1819 | 1819 |
| ta37 | 30 | 15 | 1771 | 1771 |
| ta38 | 30 | 15 | 1673 | 1673 |
| ta39 | 30 | 15 | 1795 | 1795 |
| ta40 | 30 | 15 | 1651 | 1669 |
| ta41 | 30 | 20 | 1906 | 2005 |
| ta42 | 30 | 20 | 1884 | 1937 |
| ta43 | 30 | 20 | 1809 | 1846 |
| ta44 | 30 | 20 | 1948 | 1979 |
| ta45 | 30 | 20 | 1997 | 2000 |
| ta46 | 30 | 20 | 1957 | 2004 |
| ta47 | 30 | 20 | 1807 | 1889 |
| ta48 | 30 | 20 | 1912 | 1941 |
| ta49 | 30 | 20 | 1931 | 1961 |
| ta50 | 30 | 20 | 1833 | 1923 |
| ta51 | 50 | 15 | 2760 | 2760 |
| ta52 | 50 | 15 | 2756 | 2756 |
| ta53 | 50 | 15 | 2717 | 2717 |
| ta54 | 50 | 15 | 2839 | 2839 |
| ta55 | 50 | 15 | 2679 | 2679 |
| ta56 | 50 | 15 | 2781 | 2781 |
| ta57 | 50 | 15 | 2943 | 2943 |
| ta58 | 50 | 15 | 2885 | 2885 |
| ta59 | 50 | 15 | 2655 | 2655 |
| ta60 | 50 | 15 | 2723 | 2723 |
| ta61 | 50 | 20 | 2868 | 2868 |
| ta62 | 50 | 20 | 2869 | 2869 |
| ta63 | 50 | 20 | 2755 | 2755 |
| ta64 | 50 | 20 | 2702 | 2702 |
| ta65 | 50 | 20 | 2725 | 2725 |
| ta66 | 50 | 20 | 2845 | 2845 |
| ta67 | 50 | 20 | 2825 | 2825 |
| ta68 | 50 | 20 | 2784 | 2784 |
| ta69 | 50 | 20 | 3071 | 3071 |
| ta70 | 50 | 20 | 2995 | 2995 |
| ta71 | 100 | 20 | 5464 | 5464 |
| ta72 | 100 | 20 | 5181 | 5181 |
| ta73 | 100 | 20 | 5568 | 5568 |
| ta74 | 100 | 20 | 5339 | 5339 |
| ta75 | 100 | 20 | 5392 | 5392 |
| ta76 | 100 | 20 | 5342 | 5342 |
| ta77 | 100 | 20 | 5436 | 5436 |
| ta78 | 100 | 20 | 5394 | 5394 |
| ta79 | 100 | 20 | 5358 | 5358 |
| ta80 | 100 | 20 | 5183 | 5183 |
Yamada and Nakano
| Instance | Jobs | Machines | Lower bound | Upper bound |
|---|---|---|---|---|
| yn01 | 20 | 20 | 884 | 884 |
| yn02 | 20 | 20 | 870 | 904 |
| yn03 | 20 | 20 | 859 | 892 |
| yn04 | 20 | 20 | 929 | 968 |
Referências
HOORN, J. J. van. The Current state of bounds on benchmark instances of the job-shopscheduling problem. J. Sched., Springer US, v. 21, n. 1, p. 127–128, feb 2018. ISSN 1094-6136. Disponível em: <http://link.springer.com/10.1007/s10951-017-0547-8>.
ADAMS, J.; BALAS, E.; ZAWACK, D. The Shifting Bottleneck Procedure for Job Shop Scheduling. Manage. Sci., v. 34, n. 3, p. 391 401, mar 1988. ISSN 0025-1909. Disponível em: <http://pubsonline.informs.org/doi/abs/10.1287/mnsc.34.3.391>.
LAWRENCE, S. Resource Constrained Project Scheduling. An Experimental Investigation of Heuristic Scheduling Techniques (Supplement). [S.l.]: Carnegie-Mellon University, 1984.
ADAMS, J.; BALAS, E.; ZAWACK, D. The Shifting Bottleneck Procedure for Job Shop Scheduling. Manage. Sci., v. 34, n. 3, p. 391–401, mar 1988. ISSN 0025-1909. Disponível em: <http://pubsonline.informs.org/doi/abs/10.1287/mnsc.34.3.391>.
APPLEGATE, D.; COOK, W. A Computational Study of the Job-Shop SchedulingProblem. ORSA J. Comput., v. 3, n. 2, p. 149–156, 1991. ISSN 0899-1499. Disponível em: <http://pubsonline.informs.org/doi/abs/10.1287/ijoc.3.2.149>.
APPLEGATE, D.; COOK, W. A Computational Study of the Job-Shop SchedulingProblem. ORSA J. Comput., v. 3, n. 2, p. 149–156, 1991. ISSN 0899-1499. Disponível em: <http://pubsonline.informs.org/doi/abs/10.1287/ijoc.3.2.149>.
STORER, R. H.; WU, S. D.; VACCARI, R. New Search Spaces for Sequencing Problems with Application to Job Shop Scheduling. Manage. Sci., v. 38, n. 10, p. 1495–1509, 1992. ISSN 0025-1909.
STORER, R. H.; WU, S. D.; VACCARI, R. New Search Spaces for Sequencing Problems with Application to Job Shop Scheduling. Manage. Sci., v. 38, n. 10, p. 1495–1509, 1992. ISSN 0025-1909.
Yamada, T.; Nakano, R. A genetic algorithm applicable to large-scale job-shop instances.Parallel instance solving from Nat., v. 2, n. January 1992, p. 10, 1992. Disponível em: <http://dblp.uni-trier.de/db/conf/ppsn/ppsn1992.html#YamadaN92>.
Yamada, T.; Nakano, R. A genetic algorithm applicable to large-scale job-shop instances.Parallel instance solving from Nat., v. 2, n. January 1992, p. 10, 1992. Disponível em: <http://dblp.uni-trier.de/db/conf/ppsn/ppsn1992.html#YamadaN92>.
TAILLARD, E. Benchmarks for basic sheduling problems. Eur. J. Oper. Res., v. 64, p. 278–285, 1993.
DEMIRKOL, E.; MEHTA, S.; UZSOY, R. Theory and Methodology Benchmarks for shop scheduling problems. Eur. J. Oper. Res., v. 109, p. 137–14, 1998. ISSN 03772217.
